part 1, solving using matrices and cramer's rule

Published 1 year ago • 482K plays • Length 4:11

Download video MP4
Download video MP3

Similar videos

11:23

intro to matrices
3:03

if det ( a a 1 a b b 1 b c c 1 c) = 0 and vectors (1,a,a), (1,b,b) (1,c,c) are non coplanar vectors
3:48

matrices and determinants - class 12 maths | chapter 3 | ahaguru - prof. ravishankar
11:02

a matrix determinant problem
15:14

inverse of a 3x3 matrix - (the simple way)
10:48

how to multiply matrices - quick & easy!
33:39

matrices one shot in 32 minutes⏳ | class 12 maths chapter 3 | harsh sir @vedantumath
4:30

`|[a,a^2,a^3-1],[b,b^2,b^3-1],[c,c^2,c^3-1]|=0 ` prove that `abc= i ` ...
11:31

how to find the determinant of a 3x3 matrix
18:54

determinants - problems & solved examples
4:46

prove that ` |[1,a,a^3],[1,b,b^3],[1,c,c^3]|=(a-b)(b-c)(c-a)(a b c)`
3:35

`[[b c,a-b,a],[c a,b-c,b],[a b,c-a,c]] = 3abc - a^3 - b^3 - c^3`
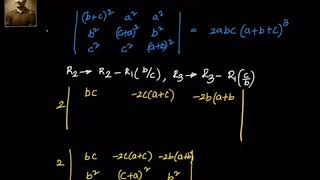12:37

matrices: determinants- prove det = 2abc(a b c)^3
$prove that : |{:(a b,b,c),(b c,c,a),(c a,a,b):}|=3 abc-a^(3)-b^(3)-c^(3) | 12 | determinants | m...$ 5:39

prove that : |{:(a b,b,c),(b c,c,a),(c a,a,b):}|=3 abc-a^(3)-b^(3)-c^(3) | 12 | determinants | m...
$prove that `|{:(1, 1, 1),(a, b, c),(a^(3), b^(3), c^(3)):}|=(a-b)(b-c)(c-a)(a b c)`$ 3:36

prove that `|{:(1, 1, 1),(a, b, c),(a^(3), b^(3), c^(3)):}|=(a-b)(b-c)(c-a)(a b c)`
5:14

problem no.2 based on 3 x 3 order determinant |ekeeda.com
0:33

solve each triangle. see examples 2 and 3 . a=9.3 cm, b=5.7 cm, c=8.2 cm
3:52

determinant solved problem - difficulty medium
3:05

show that `|[a,b,c],[a^2,b^2,c^2],[a^3,b^3,c^3]|=abc(a-b)(b-c)(c-a)`

Clip.africa.com - Privacy-policy