in exercises 19-22 , find the maximum value of |r| and any zeros of r . verify your answers numeric…
Published 2 months ago • 1 view plays • Length 0:33Download video MP4
Download video MP3
Similar videos
-
 0:33
0:33
in exercises 19-22 , find the maximum value of |r| and any zeros of r . verify your answers numeric…
-
 0:33
0:33
in exercises 19-22 , find the maximum value of |r| and any zeros of r . verify your answers numeric…
-
 4:12
4:12
calculus 1: max-min problems (20 of 30) the sum of 2 numbers
-
 6:15
6:15
calculus 1: max-min problems (8 of 30) reviewing general approach: example
-
 0:33
0:33
in exercises 1-6, use the surface integral in stokes' theorem to calculate the circulation of the f…
-
 5:20
5:20
calculus 3: triple integrals (25.5 of 25) finding the volume slice (correct answer)
-
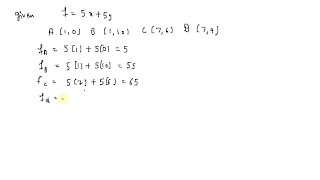 0:33
0:33
find the maximum and minimum values of each objective function over the region of feasible solution…
-
 0:33
0:33
list all possible rational zeros for the function. (enter your answers as a comma-separated list.) …
-
 10:21
10:21
comparing 999! and 500^999 in two ways
-
 10:51
10:51
a nonstandard extreme value problem
-
 8:44
8:44
sketching a derivative from the graph of a function
-
 0:33
0:33
use series to approximate the values of the integrals in exercises 19-22 with an error of magnitude…
-
 0:33
0:33
in exercises 11-22, estimate the minimum number of subintervals needed to approximate the integrals…
-
 0:33
0:33
use series to approximate the values of the integrals in exercises 19-22 with an error of magnitude…
-
 0:33
0:33
' not yet answered marked out of 10.00 question 5 find the second derivative of the following funct…
-
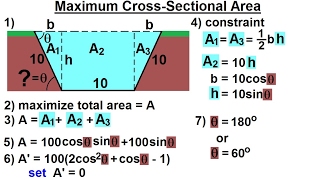 9:02
9:02
calculus 1: max-min problems (21 of 30) the maximum cross section
-
 14:20
14:20
section 3.2, ideals, 2x2 matrices over a field are simple rings
-
 0:33
0:33
in exercises 15-26, estimate the minimum number of subintervals needed to approximate the integrals…
-
 0:33
0:33
in exercises 11-22, estimate the minimum number of subintervals needed to approximate the integrals…
-
 0:33
0:33
how much heat must be absorbed by ice of mass m = 720 g at -10 oc to take it to liquid state at 15 …
-
 7:40
7:40
applications of maximisation/minimisation (1 of 2: largest rectangle area question)