prove that (n!)^2 ≤ n^n.n! for all positive integers | factorial notation | pnc | math | problem
Published 2 years ago • 2.6K plays • Length 6:25Download video MP4
Download video MP3
Similar videos
-
 3:31
3:31
prove that n^n ≥ n! for all positive integers | factorial notation | permutation and combination
-
 7:30
7:30
prove that 2^n is greater than n for all positive integers n | mathematical induction | maths
-
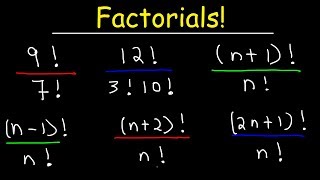 11:17
11:17
factorials explained!
-
 10:53
10:53
how to prove factorial (!)??
-
 10:45
10:45
prove by the principle of induction for all n belongs to n | 1^2 2^2 ... n^2 = n(n 1)(2n 1)/6
-
 23:49
23:49
use mathematical induction to show that 2^n is greater than n for all nonnegative integers n
-
 5:03
5:03
the secret behind -1 factorial
-
 1:58
1:58
prove that zero factorial is equal to one
-
 9:15
9:15
factorials made easy - advanced gmat number properties
-
 0:33
0:33
the factorial notation n! is defined for a positive number n as n(n - 1)(n - 2)(3)(2)(1)(0). the fo…
-
 2:58
2:58
prove n^2 n is divisible by 2 for all positive integers. principle of mathematical induction
-
 5:27
5:27
discrete mathematics : - ( factorial notation ) - 87.
-
 1:11:43
1:11:43
permutation and combination class 11
-
 8:24
8:24
|i factorial| you won't believe the outcome
-
 8:34
8:34
n!=n^3-n, a factorial equation
-
 0:45
0:45
factorial (!) ? | fun math | don't memorise
-
 8:26
8:26
how to prove that if 2^n - 1 is prime for some positive integer n, then n is also prime
-
 1:18
1:18
what is factorial n! and what to do with 0!
-
 8:18
8:18
a factorial equation | m! 12=n^2