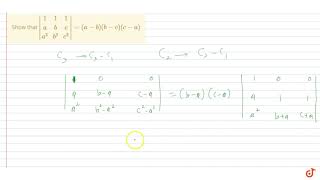
show that ` |[1,a,a^2],[1,b,b^2],[1,c,c^2]|=(a-b)(b-c)(c-a) `
Published 4 years ago • 18K plays • Length 3:32
Download video MP4
Download video MP3
Similar videos
-
![show that ` |[1,a,a^2],[1,b,b^2],[1,c,c^2]|=(a-b)(b-c)(c-a) `](https://i.ytimg.com/vi/qVrZK4vrywA/mqdefault.jpg) 3:33
3:33
show that ` |[1,a,a^2],[1,b,b^2],[1,c,c^2]|=(a-b)(b-c)(c-a) `
-
![by using properties of determinants, show that: (i) |[ 1 a a^2; 1 b b^2; 1 c c^2 ]|=(...](https://i.ytimg.com/vi/YCJtXVpHIlQ/mqdefault.jpg) 9:08
9:08
by using properties of determinants, show that: (i) |[ 1 a a^2; 1 b b^2; 1 c c^2 ]|=(...
-
 5:50
5:50
to prove |1 a a^2,1 b b^2,1 c c^2| equal to (a-b)(b-c )(c-a)
-
 2:26
2:26
show that `|(1,1,1), (a,b,c),(a^2,b^2,c^2)|=(a-b)(b-c)(c-a)`
-
 6:42
6:42
by using properties of determinants. show that:(i) `|1a a^2 1bb^2 1cc^2|=(a-b)(b-c)(c-a)`(ii) `|1 1
-
 2:50
2:50
the value of the determinant `|{:(1,a, a^(2)-bc),(1, b, b^(2)-ca),(1, c, c^(2)-ab):}|` is�..
-
 5:30
5:30
using properties of determinant, prove that `abs{:(1,a,a^2),(1,b,b^2), | class 12 maths | doubtnut
-
 4:06
4:06
laq video 8 || determenent problems || find the det of =(a-b)(b-c)(c-a)
-
 8:19
8:19
how to solve determinants using properties of determinants || matrices and determinants || part 5
-
 1:33
1:33
expansion of (2x 1)² || a plus b whole square || (a b)²=a² 2ab b² ||algebraic identity
-
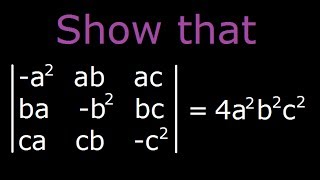 5:27
5:27
simplify determinant using properties
-
 6:41
6:41
xii determinants prove that a^2 1 ab ac ab b^2 1 bc ca cb c^2 1 = 1 a^2 b^2
-
 4:32
4:32
how to solve determinants question using properties of determinants|matrices and determinant|proof|
-
![`([1,1,1],[a,b,c],[a^2,b^2,c^2])=(a-b)(b-c)(c-a)`](https://i.ytimg.com/vi/jMbj2UGKMP0/mqdefault.jpg) 8:08
8:08
`([1,1,1],[a,b,c],[a^2,b^2,c^2])=(a-b)(b-c)(c-a)`
-
![show that `|[1,1,1],[a,b,c],[bc,ca,ab]|=(a-b)(b-c)(c-a)`](https://i.ytimg.com/vi/xq2HElnyzh4/mqdefault.jpg) 2:39
2:39
show that `|[1,1,1],[a,b,c],[bc,ca,ab]|=(a-b)(b-c)(c-a)`
-
 2:32
2:32
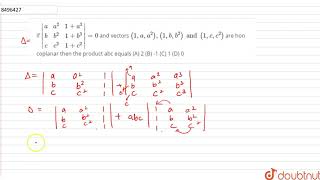
if `|(a,a^2,1 a^3),(b,b^2,1 b^3),(c,c^2,1 c^2)|=0` and vectors `(1,a,a^2),(1,b,b^2) and (1,c,c^2)`
-
![show that|[1 a,1,1],[1,1 b,1],[1,1,1 c]|=abc(1 1/a 1/b 1/c)=abc bc ca ab | class 12 | determinan...](https://i.ytimg.com/vi/ETIQRVzEd_M/mqdefault.jpg) 4:35
4:35
show that|[1 a,1,1],[1,1 b,1],[1,1,1 c]|=abc(1 1/a 1/b 1/c)=abc bc ca ab | class 12 | determinan...
-
 3:03
3:03
if det ( a a 1 a b b 1 b c c 1 c) = 0 and vectors (1,a,a), (1,b,b) (1,c,c) are non coplanar vectors
-
 5:37
5:37
by using properties of determinants show that 1 a^2-b^2 | using determinant prove that (1 a^2 b^2)^3
-
![using the properties of determinants show that `[[1,1,1],[a^2,b^2,c^2], | class 12 maths | doubtnut](https://i.ytimg.com/vi/mwCYnIcOZg4/mqdefault.jpg) 8:12
8:12
using the properties of determinants show that `[[1,1,1],[a^2,b^2,c^2], | class 12 maths | doubtnut
-
 4:25
4:25
if `|{:(a,,a^(2),,1 a^(3)),(b,,b^(2),,1 b^(3)),(c,,c^(2),,1 c^(3)):}|=0` and the vectors `overset
-
 3:36
3:36
prove that `|{:(1, 1, 1),(a, b, c),(a^(3), b^(3), c^(3)):}|=(a-b)(b-c)(c-a)(a b c)`
Clip.africa.com - Privacy-policy
![show that ` |[1,a,a^2],[1,b,b^2],[1,c,c^2]|=(a-b)(b-c)(c-a) `](https://i.ytimg.com/vi/qVrZK4vrywA/mqdefault.jpg) 3:33
3:33
![by using properties of determinants, show that: (i) |[ 1 a a^2; 1 b b^2; 1 c c^2 ]|=(...](https://i.ytimg.com/vi/YCJtXVpHIlQ/mqdefault.jpg) 9:08
9:08
 5:50
5:50
 2:26
2:26
 6:42
6:42
 2:50
2:50
 5:30
5:30
 4:06
4:06
 8:19
8:19
 1:33
1:33
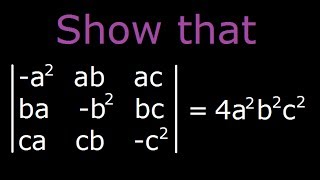 5:27
5:27
 6:41
6:41
 4:32
4:32
![`([1,1,1],[a,b,c],[a^2,b^2,c^2])=(a-b)(b-c)(c-a)`](https://i.ytimg.com/vi/jMbj2UGKMP0/mqdefault.jpg) 8:08
8:08
![show that `|[1,1,1],[a,b,c],[bc,ca,ab]|=(a-b)(b-c)(c-a)`](https://i.ytimg.com/vi/xq2HElnyzh4/mqdefault.jpg) 2:39
2:39
 2:32
2:32
![show that|[1 a,1,1],[1,1 b,1],[1,1,1 c]|=abc(1 1/a 1/b 1/c)=abc bc ca ab | class 12 | determinan...](https://i.ytimg.com/vi/ETIQRVzEd_M/mqdefault.jpg) 4:35
4:35
 3:03
3:03
 5:37
5:37
![using the properties of determinants show that `[[1,1,1],[a^2,b^2,c^2], | class 12 maths | doubtnut](https://i.ytimg.com/vi/mwCYnIcOZg4/mqdefault.jpg) 8:12
8:12
 4:25
4:25
 3:36
3:36